Introduction
Space closure requires a solid comprehension of biomechanics in order to avoid undesirable side effects.1 Infact it is one of the most challenging procedure. To move teeth in a controlled fashion and achieve optimum results, various factors should be considered including correct mechanical principles, appropriate interplay between the orthodontic appliance and anatomic structures and the efficacy of tooth movement.2 The 2 methods of space closure include
In general, six goals should be considered for space closure:
Differential space closure-anchorage control.
Minimum patient cooperation.
Axial inclination control.
Control of rotations and arch width.
Optimum biological response.
Operator convenience.3
Closing loops are used in orthodontics with both segmental and continuous arch wires to generate the desired forces and moments to move teeth in a predictable manner. Since they are frictionless, the force generated by loops can be applied directly to a tooth or a group of teeth thereby preventing unwanted resistance. 4
Storey and Smith, Reitan and Krishnan and Davidovitch recommended the use of light forces, while Oppenheim and Burstone suggested the use of light forces and, whenever possible, continuous forces. 5
The spring characteristics of the closing loops are determined by:
Wire material,
Archwire cross-section,
Inter-bracket distance,
Configuration,
Position of the loop. 3
The three primary characteristics (Spring characteristics in-turn affect these primary characteristics) of a retraction spring are:
The moment/force ratio which determines the centre of rotation of tooth during its movement.
The greatest force at yield that can be delivered from a retraction spring without permanent deformations.
Load to deflection rate.6
The moment-to-force ratio is probably the most important characteristic of a retraction arch-wire. Simplicity of fabrication and delivery is always secondary to Low load/deflection, efficiency and space closure control.
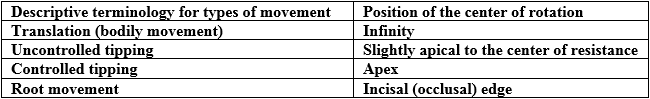
What is moment to force ratio
The type of movement exhibited by a tooth is determined by the ratio between the magnitude of the couple (M) and the force (F) applied at the bracket.7
M/F ratio for translation – 10:18
At these relatively high moment-to-force ratio levels, stresses reportedly distribute more evenly through the entire root with minimal changes in the mechanical properties during activation; this reduces injuries to teeth and surrounding tissues.4
Configuration of the loop – As the wire material increases between the brackets, the load deflection rate decreases. This indicates that if the loop height increases, there is a reduction in load deflection rate and there is an increase in the moment to force ratio.
Moment of loops can be classified into 2 sub headings
Inherent moment is the one present in the loop because of the loop design. The design of the loop determines the amount of inherent moment. An ideal loop is one that has high inherent moment and low activation moment.
Loop Height: As the loop height increases, the moment-to-force ratio increases. Burstone and Koenig reported that a 6- mm-high vertical loop had a moment-to-force ratio of approximately 2, whereas a 10-mm-high vertical loop had a moment-to-force ratio of about 4 for a 7-mm horizontal loop length. 4 Unfortunately, no loop can reach a moment-to-force ratio greater than its height.
Loop Positioning: Off-centre positioning is also used to modify the moment-to-force ratio. Faulkner et al evaluated that T-loops placed off- centred produced a different moment to force ratio with an increase in moment produced on the bracket which is closer to the loop. 9
Reduction in the force level and increase in moment required for root control can also be achieved by increasing the
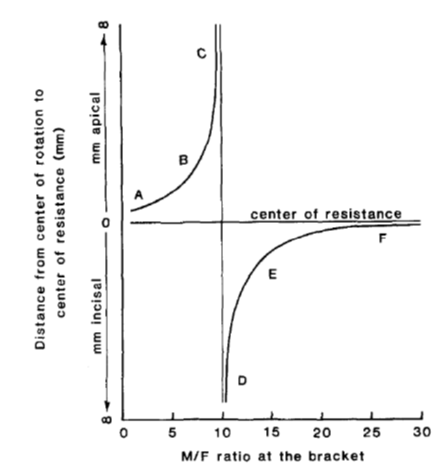
Activation moment refers to the one that is incorporated by the addition of activation and pre-activation bends. The activation bends increases the moment to force ratio but has the disadvantage that it deforms the original loop configuration. Gable bends are given to increase the root control, avoiding ‘dumping’ of teeth into the extraction space. The degree of pre-activation bends can also change the anchorage also.6
Hence while closing a space with loops, re-activation of the loops should not be done immediately after the space is closed because, initially, the space closure occurs by controlled tipping followed by bodily movement and finally root movement. Once all the 3 phases of movement are completed, the re-activation is to be done. Hence, a time period of 4 weeks should be the gap between activation of loops.
A perfect example of one such loop is T-loops - Initially after pre-activation, controlled tipping occurs (M/F 8:1), as space closes and spring deactivates, the force level decreases so translation occurs (10:1), further deactivation leads to root movements (12:1).10
Figure 3
The moment present in A is the inherent moment while that present in the B after pre-activation bends is both inherent and residual moment. 11
The results of the study done by Faulkner et al clearly showed that the ordinary vertical loop, even with the modifications outlined, is limited in it’s ability to produce M/F ratios that approach those necessary for translation or even controlled tipping. The largest M/F ratio was less than 4 mm and was essentially constant over a very limited activation range (0 to 1.5 mm). Hence, pre-activation bends were incorporated to increase the moment to force ratio to desirable levels. 12 But this produced the risk of deforming the loop design, thus the amount of activation could not be determined leading to indeterminate force systems.
Importance of OPUS Loops
Introduced by Siatkowski, this loop has an L shape with a helix in the apical portion of the L to increase the moment-to-force ratio. Siatkowski reported that the Opus 70 loop, with the vertical legs tipped 700 backward, had a moment-to-forceratio as high as 8.7 mm, which was higher thancould be obtained by vertical loops or T-loops with similar dimensions. 4
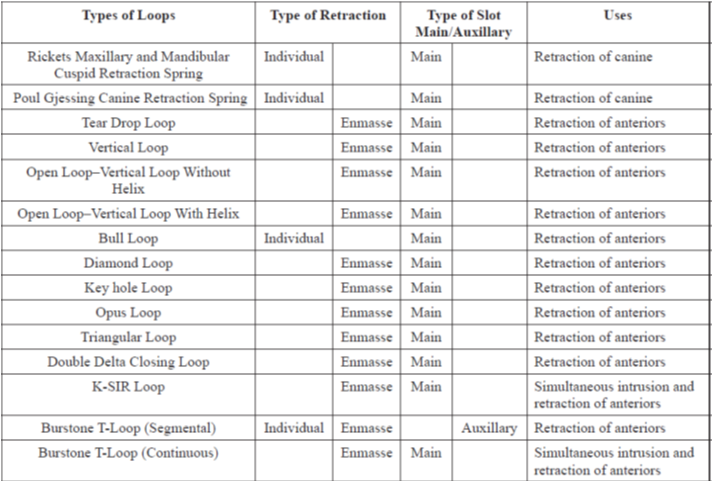
Advantages of Frictionless Mechanics
Conclusion
A thorough understanding of the M/F ratio is required for the correct clinical application of the loops. Frictionless mechanics possess greater advantages if properly used. But minor error in mechanics can result in major errorsin tooth movement. Various studies have showed that Friction mechanics were superior to frictionless mechanics for arch dimensional maintenance and rotational control. Frictionless mechanics were more effective than friction mechanics at reducing the tipping and extrusion.12 Each technique has its own advantages and disadvantages, but the clinician with his knowledge and skills can make each technique effective.
Article
DOI
